Dimensional Formula of Potential Energy – Detailed Explanation
Explore the dimensions of energy, a fascinating dimension. Discover what is the dimensional formula for potential energy and demystify complex concepts so that everyone with a curiosity can understand them.
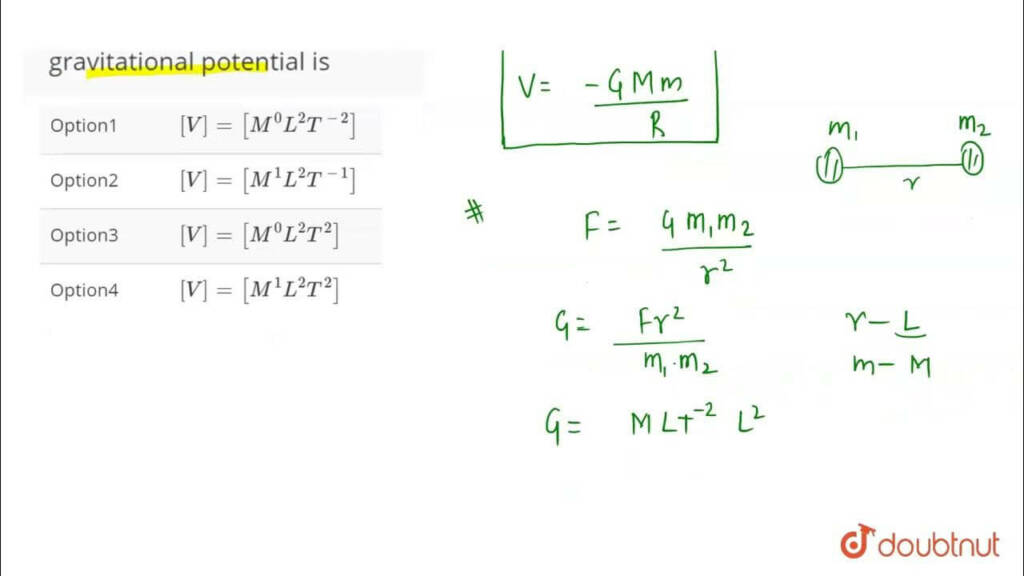
Dimensional Formula of Potential Energy
Today, let us discuss the “Dimensional Formula of Potential Energy,” which is a really fascinating topic. Now, the concept of potential energy may seem overwhelming and complex, but don’t worry! I’ll make it incredibly easy for you.
What is a potential energy?
Let’s start by explaining what potential energy is. Now imagine a bouncy ball elevated on a high shelf. It’s not moving, but when it does fall, it might bounce very high. Potential energy is what we refer to as that stored energy.
Dimensional Formula of Potential Energy with Explanation
Now, the “Dimensional Formula” is simply a sophisticated method of figuring out the units of measurement. ‘Joules’ (J) is the unit we use for potential energy. It is comparable to measuring weight or length in grams or centimeters. Therefore, [M1L2T-2] is the dimensional formula for potential energy, where M is for mass, L is for length, and T is for time.
Also Read: What Is Bulk Posting In SBI? – Detailed Answer and Process
What does that mean, though? It indicates that the mass of the object, its maximum fall height, and the duration of the fall are the three factors that determine potential energy. Remember that an object has more potential energy the heavier and higher it is. Additionally, it can acquire more potential energy the longer it takes to fall.
Thus, consider the potential energy a ball or a swing at the park has the next time you see it. And keep in mind that knowing the dimensional formula can assist us in calculating and comprehending the amount of potential energy that is contained in various objects in our environment.